What is a Shear field?

This article aims to cover the theoretical background of the shear field stiffness determination methods implemented in Consteel. Modeling with the shear field stiffness based method will also be compared with shell modeling of trapezoidal deckings in Consteel.

Theoretical background

Modeling the shear stiffness of trapezoidal deckings is used to utilize their contribution to stabilizing the main structure. The possibility to consider the shear stiffness of sheetings is implemented at finite element level in Consteel and ensures easy modeling through its application onto beam elements.

Shear panel definitions

For the discussion let’s establish some basic definitions regarding shear panels.

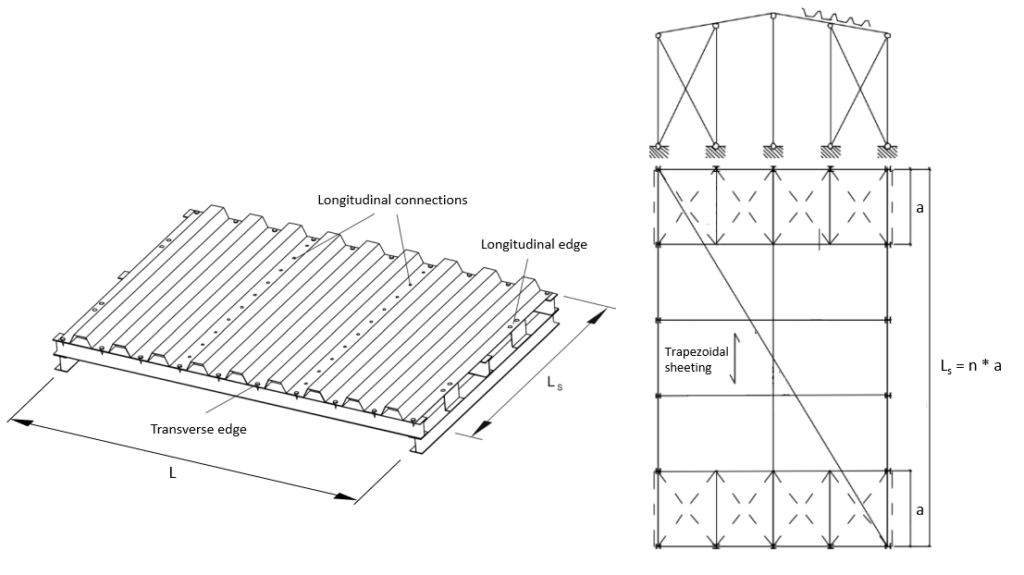
Dimensions:
- L [m]: width of the shear field, also the span of the stabilized beam
- L_s [m]: complete length of the shear field parallel to the ribs
- a [m]: effective shear field length for only one connecting beam element
Stiffnesses:
- G_s [kN/m]: specific shear stiffness considering a 1 m long strip of an “L_s“ long shear field
- S [kN]: shear stiffness of the complete shear field

Determaination of the shear field stiffness

The general formula to calculate the shear stiffness in Consteel is the following:

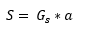
There are 4 methods implemented in Consteel to determine the shear field stiffness:

Schardt/Strehl method: (K1, K2), DIN 18807-1:1987-06 [1]

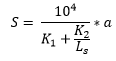
improved Schardt/Strehl method: (K1, K2, K1*, K2* and e_L)

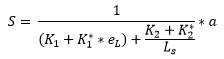
Bryan/Davies method: (K1, K2, K1*, K2*, e_L, α₁, α₂, α₃, α₄)

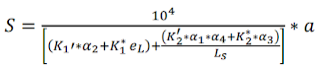
Eurocode 3: (1993-1-3 10.1.1 (10)) [2]

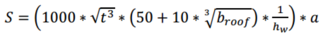
See in more detail here: Determination of shear field stiffness and application in Consteel

The first 3 methods are based on the 1. one, the Schardt/Strehl method. These methods operate on the same principle by calculating the shear stiffness from values (K₁, K₂, etc.) provided by the manufacturer of the sheetings. The 2. and 3. methods are more developed versions of the 1. one, trying to more accurately calculate the shear stiffness by introducing additional parameters to account for more sources of the overall shear stiffness of the sheeting.

The 4. method that is found in Eurocode 3 can be more generally applied since that doesn’t require such product specific values.

A basic assumption in case of these methods is that the sheeting is connected at every rib to the beams that it stabilizes (purlins in most cases). An additional modifying factor in case of all these methods is if the trapezoidal sheeting is not fixed at every rib, but every second rib, then the final “S” shear stiffness should be substituted by 0,2*S.

Theoretical background of the Schardt/Strehl method

This approach is based on a model assuming a fully linear elastic behaviour of the diaphragm. The ultimate limit state is therefore defined by yielding in the corner radius at the flange-web transition. The mechanical model also assumes that the sheeting is fixed to the substructure at all 4 edges. Shear forces R_Q and R_L are acting on the sheeting at the individual fixed points on the lower flanges where the sheeting is screwed to the substructure. The number of waves in the sheeting is assumed to be large enough so that the individual forces acting at the transverse edges in the middle are assumed to be constant (n>10). The length “L_s” of the shear field can be arbitrary, but should be in reasonable proportion to the width “L” of the shear field (<4).

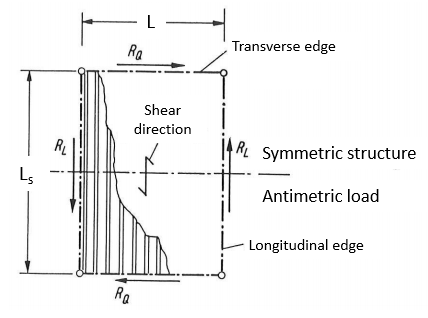
Based on these assumptions the mechanical analysis can be isolated to a half of one wave of the sheeting as shown on the left-hand side of the following figure:

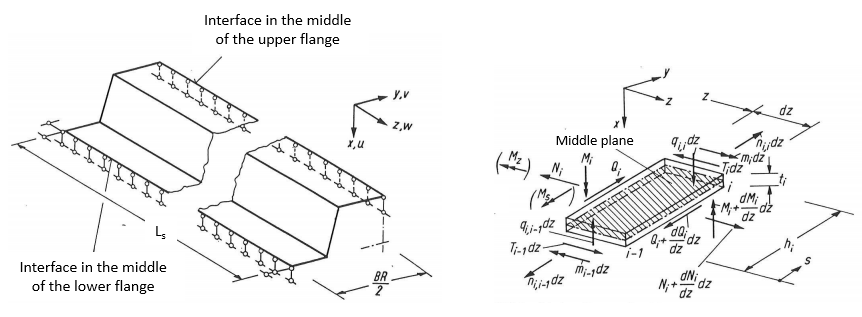
On the right-hand side the considered internal forces are shown for one slice of the sheeting.

Assumptions for the mechanical model:

The M_s and M_z moments are neglected (shown in brackets on the right-hand side figure).
Transverse bending moments “m_i” at the level of the plate are considered. These moments have a 0 value in the center of the lower and upper flanges.
The longitudinal stresses σ_z are constant over the thickness “t_i” of the plates and linearly distributed over the height “h_i”.
The flexibility of connections is neglected.

The method accounts for the following effects:

Shear deformation: corresponding value: K₁ [m/kN] shows the sheetings compliance coming from shear deformation. The lower this value is, the more stiffness the sheeting has.
Warping deformation: corresponding value: K₂ [m²/kN] shows the sheetings compliance coming from warping deformation. The lower this value is, the more stiffness the sheeting has.

Component considering shear deformation

The value K₁ can be calculated from the following formula based on the properties of the trapezoidal sheeting:

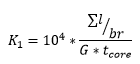
where

∑l [mm]: Summed up length of all the plates within one full wave
br [mm]: length of one complete wave
G [N/mm²]: shear modulus
t_core [mm]: structural thickness. (generally: t_core = t_nominal - 0,04 mm)

The formula of the K₁ value is similar to how it should be calculated in case of a planar plate, but its thickness corrected with the ∑l/br ratio, or in other words the ratio of the complete length of the plates to the length of one wave. The K₁ shear deformation compliance value is directly proportional to the ∑l/br ratio, therefore if a certain trapezoidal sheetings height is increased with everything else left the same, the corresponding K₁ value would increase, and the stiffness coming from shear deformation would decrease. On the other hand the K₁ value is inversely proportional to “G” shear modulus and “t_core“ structural thickness, so if either of these values would increase, K₁ would decrease, and the stiffness coming from shear deformation would increase.

Component considering warping deformation

The K₂ parameter further softens the structure taking into account the warping deformations. The detailed calculation of the K₂ parameter will not be shown here due to its extensiveness and complexity. The details of this calculation can be found in the literature [5]. The calculation is based on the “Folded Plate” theory [8]. To obtain the K₂ parameter the warping displacements and warping coordinates have to be calculated for the sheeting. The following figure shows an example for the normalized warping displacements and deformed shapes for k=1 and k=2:

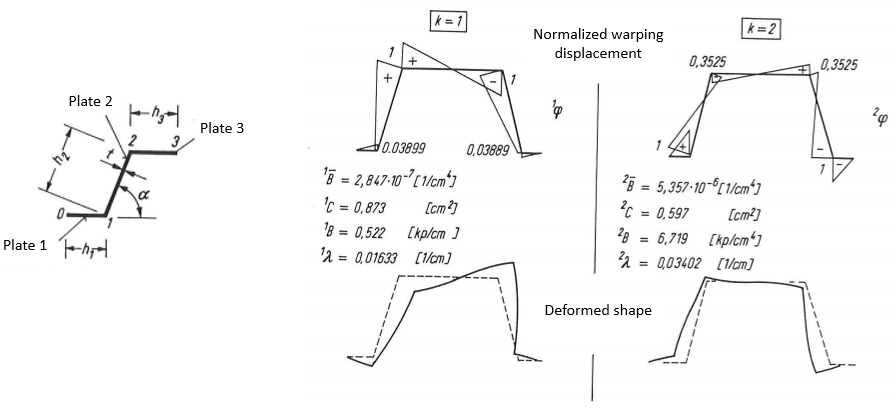
k=1 and k=2 are connected to individual solutions for the differential equation system describing the mechanical behavior based on the “Folded Plate” theory.

Calculating shear stiffness

The behavior of the two components in the formula of the shear stiffness is different. The part that considers the shear deformation only depends on the effective width “a”, but independent from the total length of the shear field “L_s”. On the other hand the part that considers the warping deformation is also dependent on the total length of the shear field “L_s”. The K₂ parameter in the denominator is divided by L_s, which means that the longer the total shear field is, the larger the specific shear stiffness is going to get because of the contribution of the warping deformations.

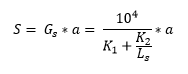
Also if the total length of the shear field “L_s“ would get really low, then K₂/L_s would approach infinity, which means that the stiffness approaches zero. For this reason a minimal length for sheetings “L_s,min” is also provided by the manufacturers, which gives the specific shear stiffness “G_s” a minimum value.

Comparison against shell models

The effect of the shear stiffness of a trapezoidal sheeting can be modeled in multiple ways. In Consteel additionally to built-in shear field field object applicable on beam elements, the sheeting can be modeled directly with shell elements. This latter approach is more complicated and time consuming to set up, but should provide similar results. Such a comparison was prepared in Consteel.

Examined structure

Stabilized beam: IPE300 S235

Span: L = 4140 mm

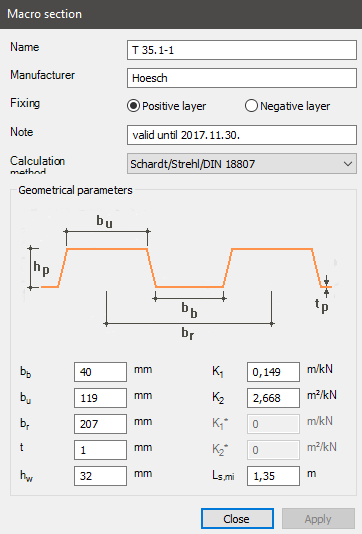
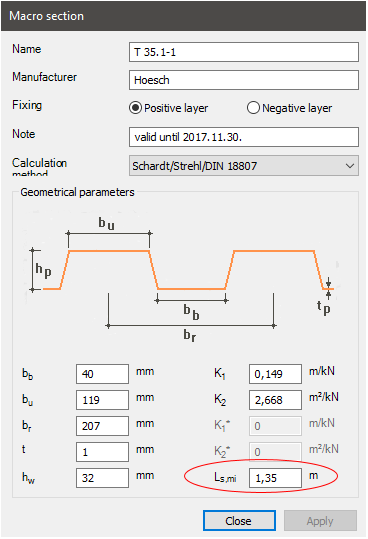
Type of trapezoidal sheeting: Hoesch T 35.1

Examined thicknesses: 0,75 mm; 1 mm; 1,25 mm
Examined sheeting lengths: 2 m, 3 m

Horizontal line load: q_y = 10 kN/m

The load and the trapezoidal sheeting are both acting on the centerline of the stabilized beam.

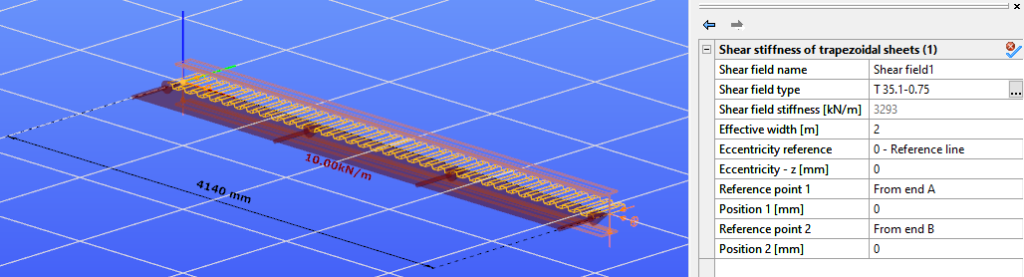
Consteel shear field model

In this modeling version the stabilizing effect of the trapezoidal sheeting is modeled by the shear field object implemented into Consteel.

Example figure: L_s = 2 m, a = 2 m, G_s = 3293 kN/m, S = 6586 kN

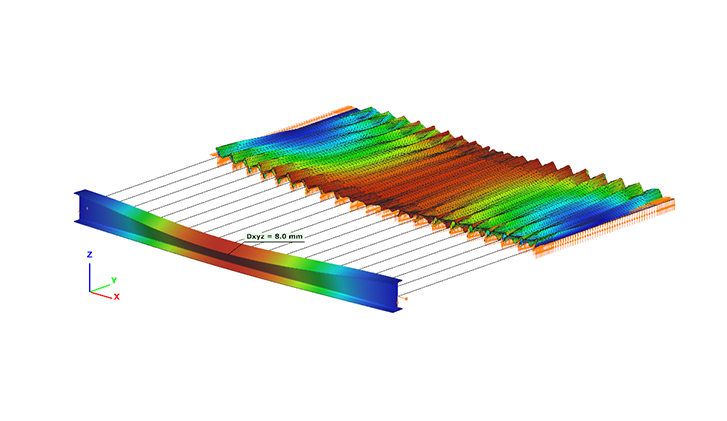
Consteel shell model

In this modeling version, the trapezoidal sheeting is modeled by shell elements. The thicknesses of the shell elements are equal to the structural thickness t_core. The model of the trapezoidal sheeting is included in a frame made from beam elements. The sheeting is connected to the frame by link elements at the lower flanges where the sheeting is screwed to the substructure. The frame is included in the model in order to connect the shell elements to the main beam. The beams of the frame have a cross-section that has relatively insignificant weaker axis inertia compared to the shear stiffness of the sheeting. The shell elements are connected through the frame to the main beam by link elements that only transfer force in their axial direction.

Example figure: L_s = 2 m, a = 2 m

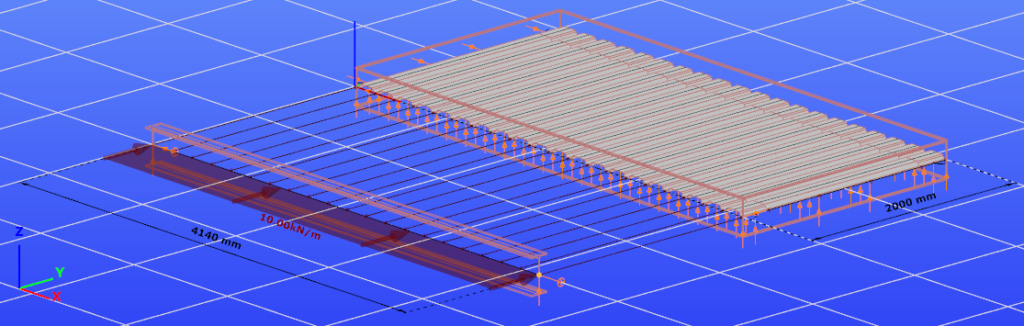
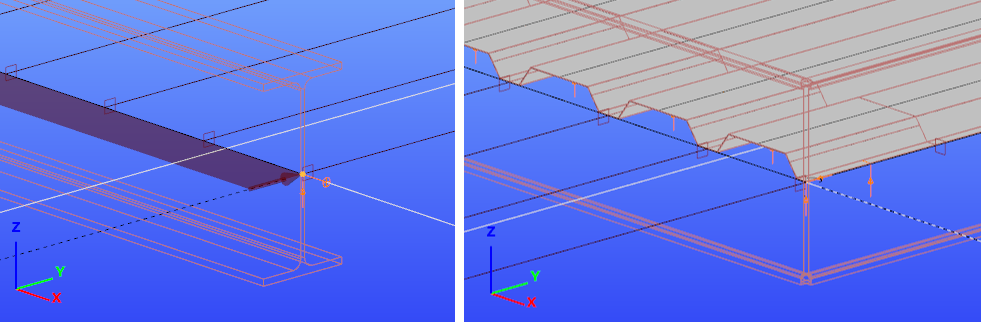
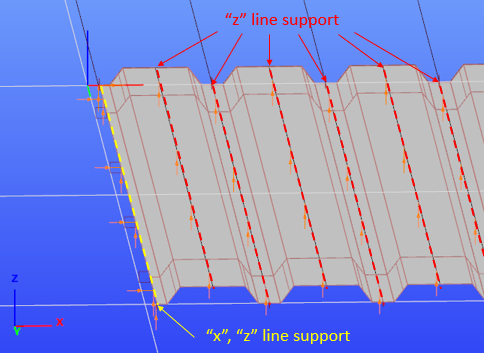
The shell elements are also supported in the vertical direction along the middle lines of its top and bottom flanges in order to eliminate the bending deformation resulting from the eccentric compression load on the sheeting, since the shear field model also does not take this effect into account. The edges on both sides of the sheeting are supported against “x” and “z” directional displacements in order to account for the sheeting being fixed to the substructure at all 4 of its edges. The line supports on the plate elements are shown on the following picture viewing the structure from below.